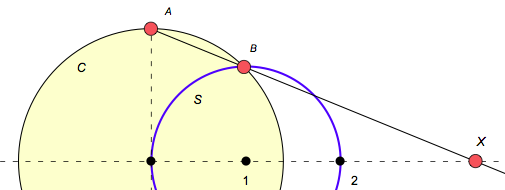
Problem of the Week 1192

A Tale of Two Circles

Let S be the circle of radius 1 centered at (1, 0).

Consider a circle C centered at the origin and let A and B mark the intersection of C's upper half with the positive y-axis and S. Extend the line AB rightward, letting X be its x-intercept.

What happens to X as C becomes smaller and smaller?

© Copyright 2014 Stan Wagon. Reproduced with permission.

Source: Shmuel Avital and Ed Barbeau, Nonintuitive solutions to problems, For the Learning of Mathematics 11:3 (1991) 2-18.

[View the solution]